At the end of 30 months, copper prices will have increased by what percentage over today’s price?

The following question requires your selection of CCC/CCE Scenario 4 (2.7.50.1.1) from the right side of your split screen, using the drop down menu, to reference during your response/choice of responses.

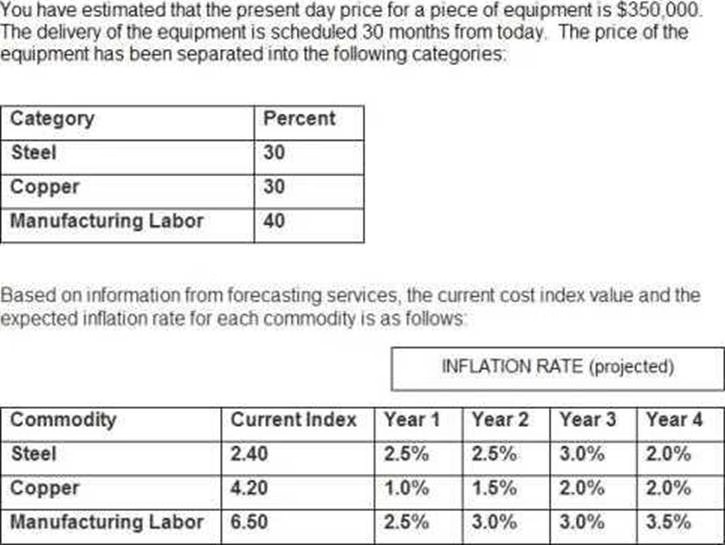
At the end of 30 months, copper prices will have increased by what percentage over today’s price?
A . 4.6%
B . 3.5%
C . 6.6%
D . 2.5%

View Answer

Answer: C

Explanation:

To calculate the increase in copper prices over 30 months (which is 2.5 years), we apply the inflation rates for the first two years and half of the third year:

Year 1: 1.0%

Year 2: 1.5%

Year 3 (half-year): 1.5% / 2 = 0.75%

The total percentage increase is calculated as:

Total Increase=(1+0.01)×(1+0.015)×(1+0.0075)−1text{Total Increase} = (1 + 0.01) times (1 + 0.015) times (1 + 0.0075) – 1Total Increase=(1+0.01)×(1+0.015)×(1+0.0075)−1 text{Total Increase} = 1.01 times 1.015 times 1.0075 – 1 approx 1.066 – 1 = 0.066 text{ or 6.6%}

So, the correct answer is C. 6.6%.